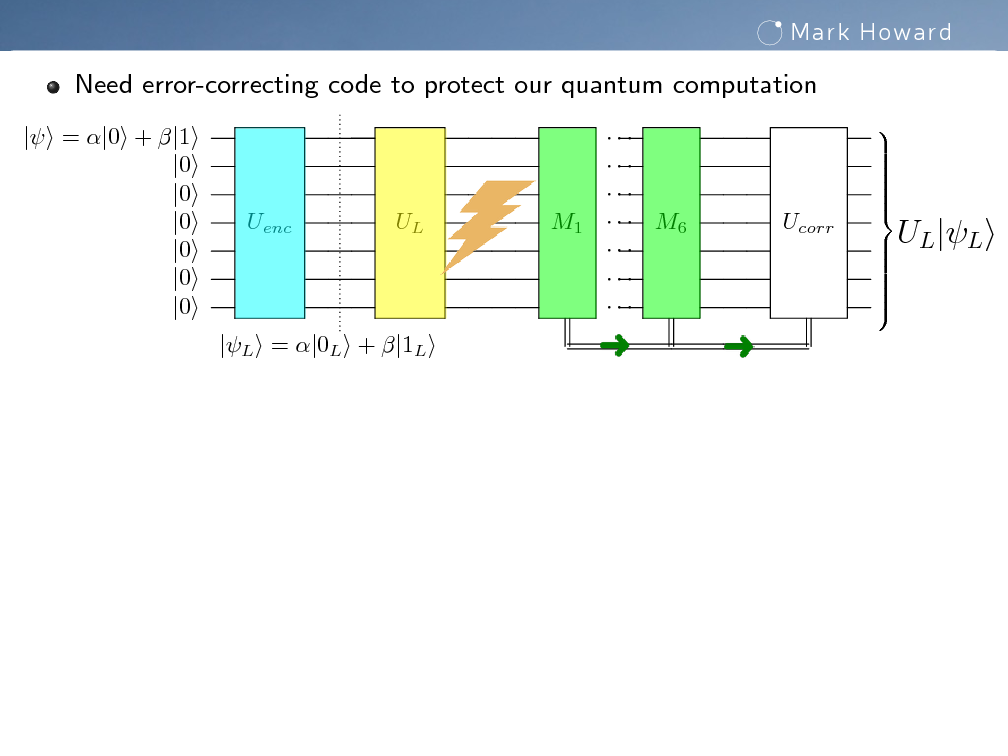
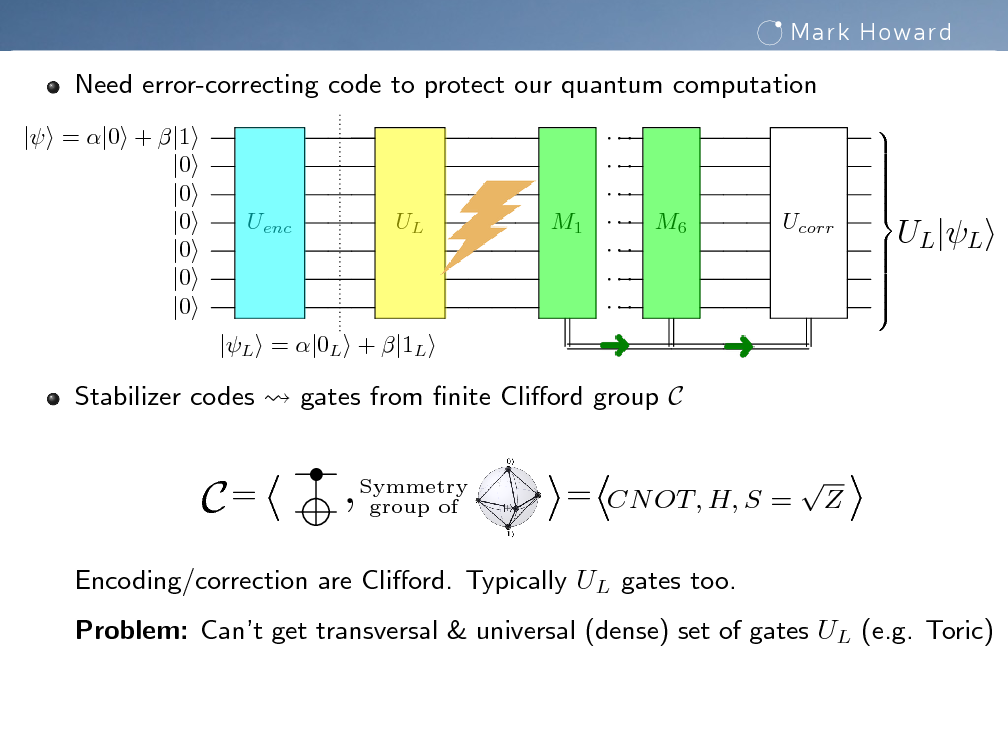
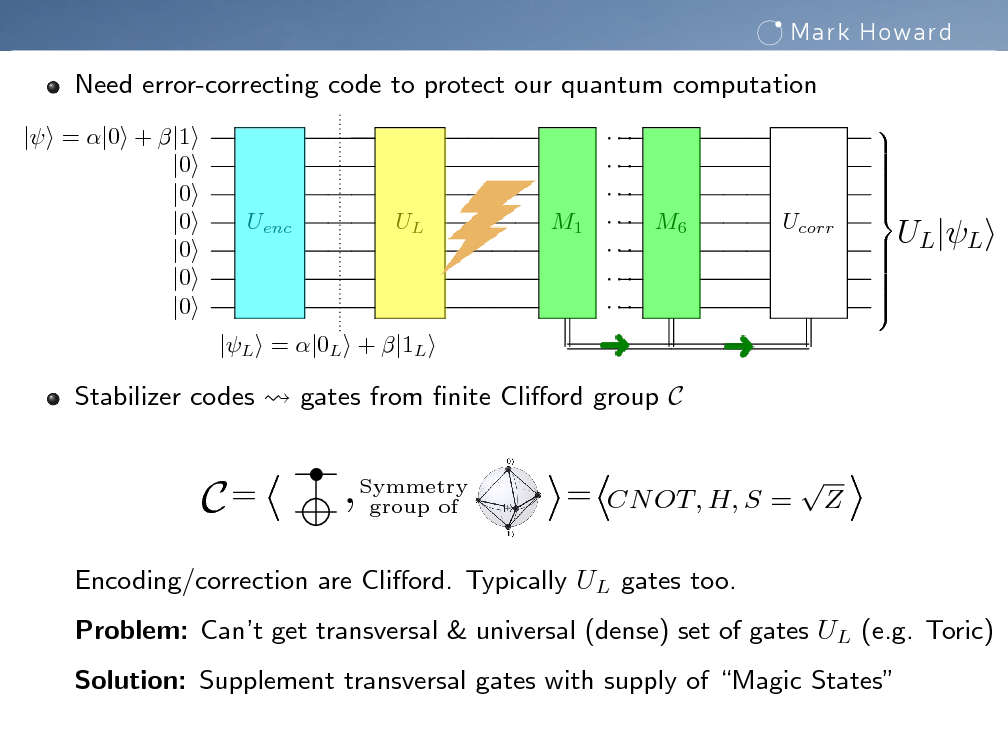
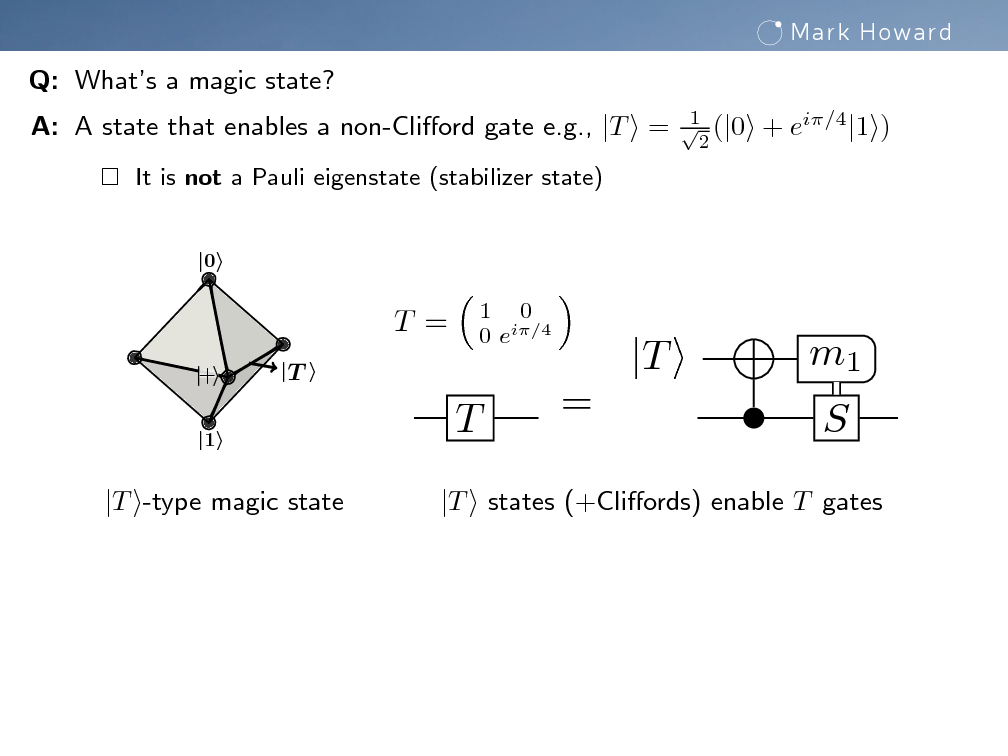
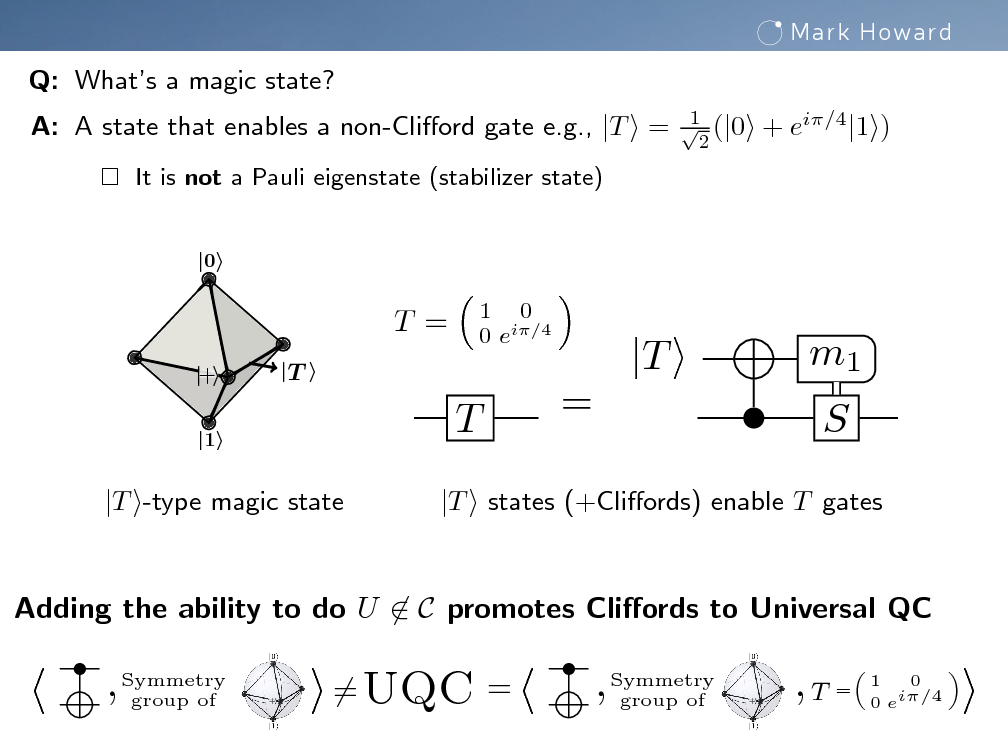
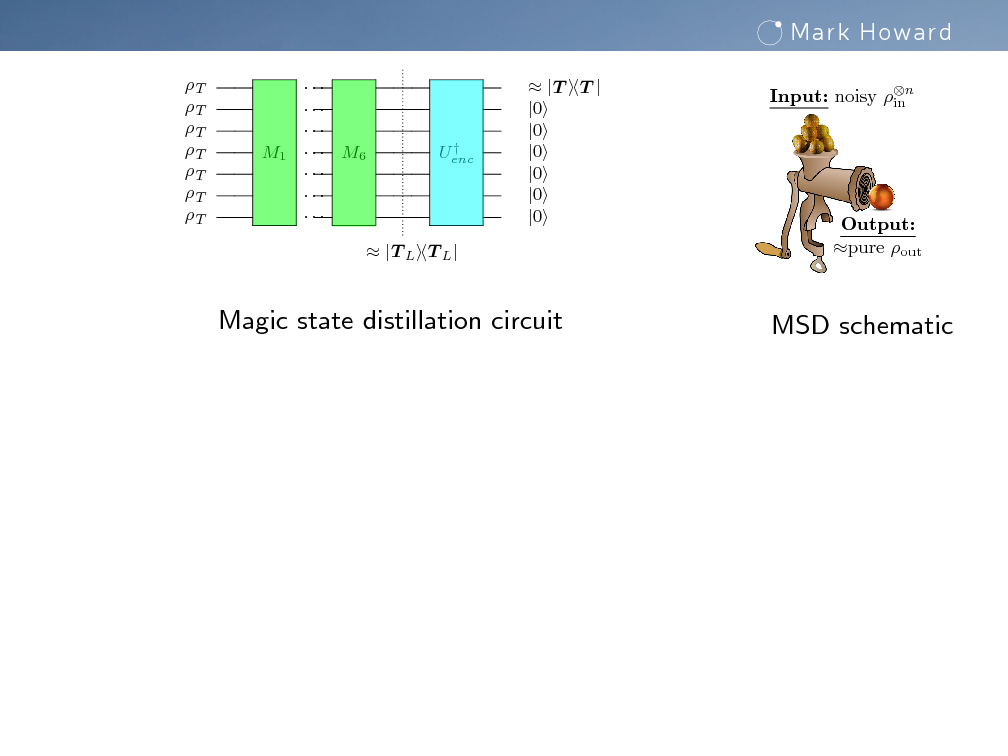
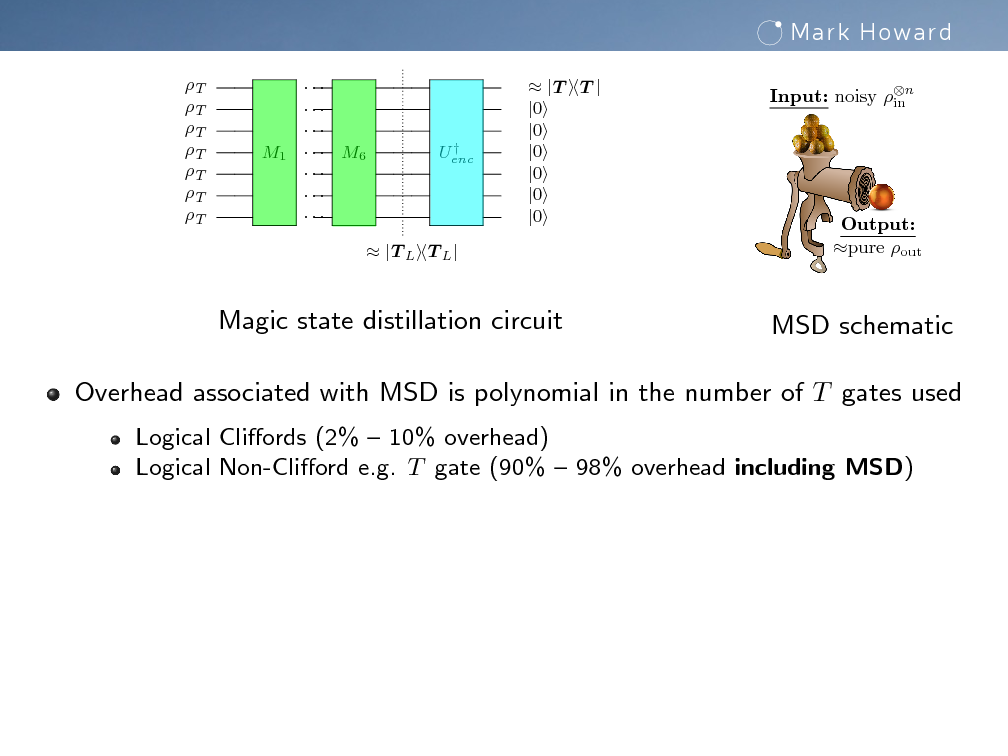
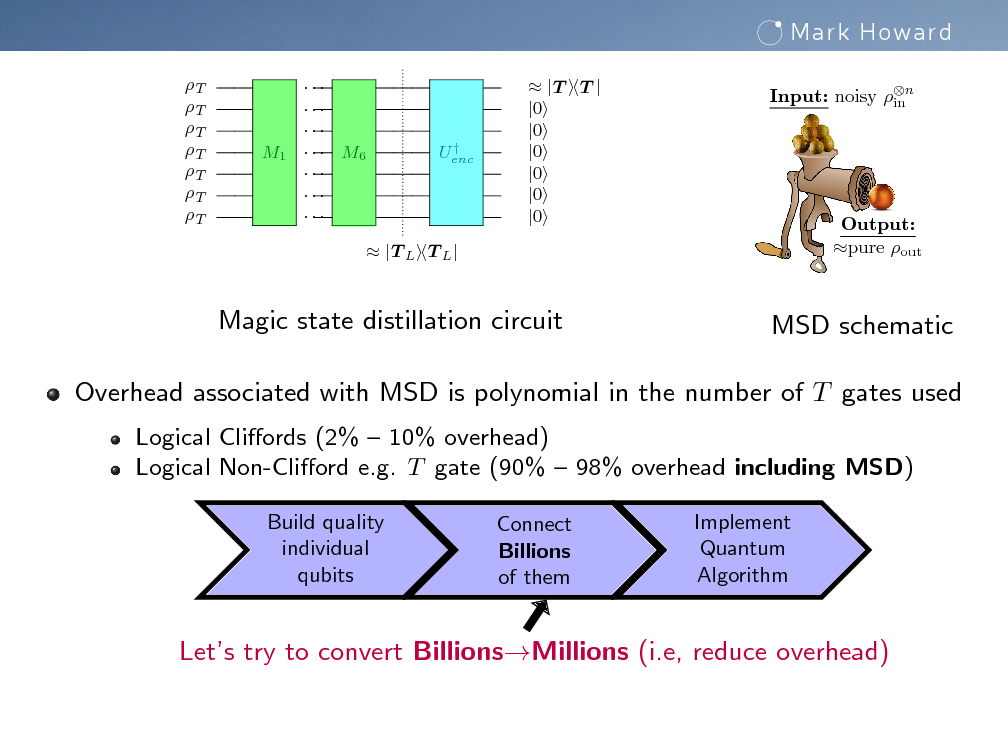
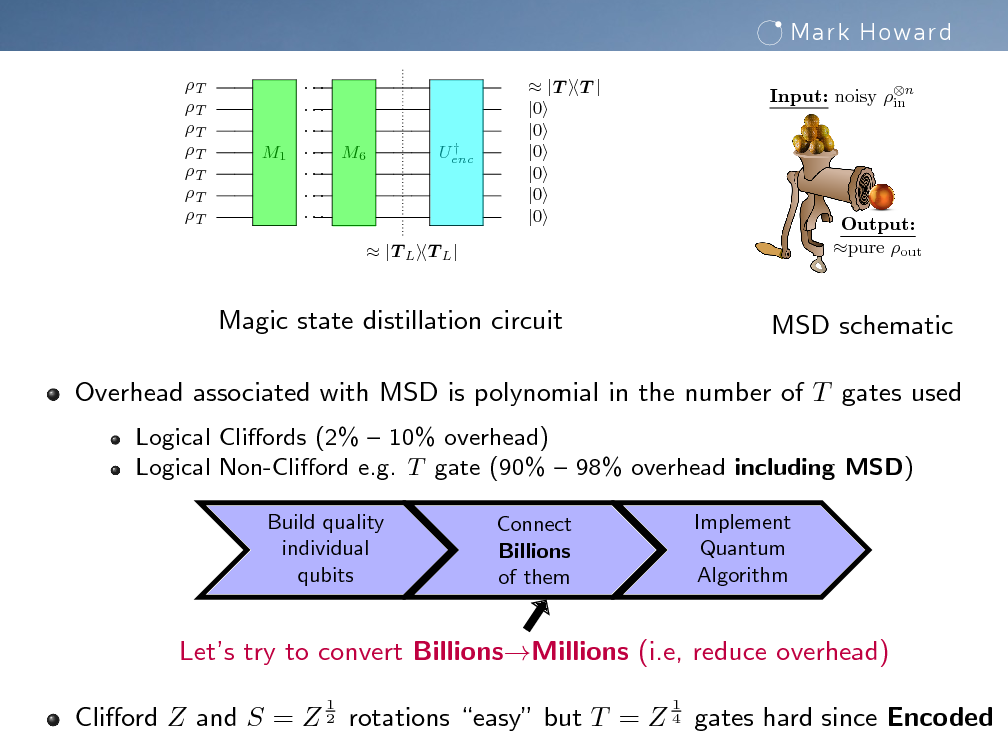
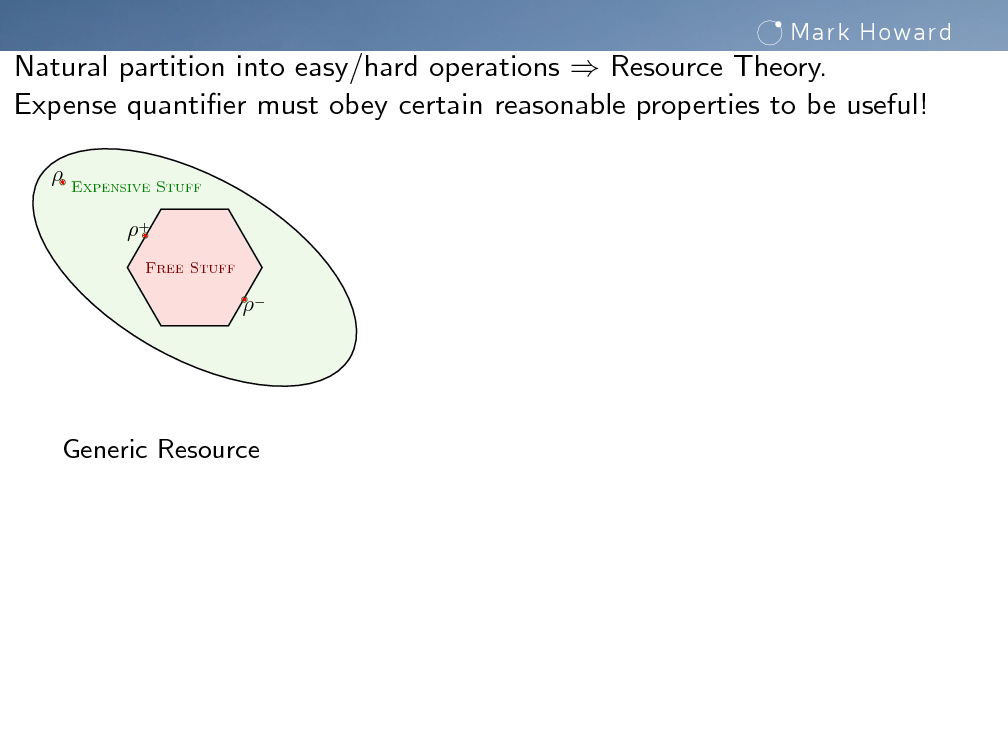
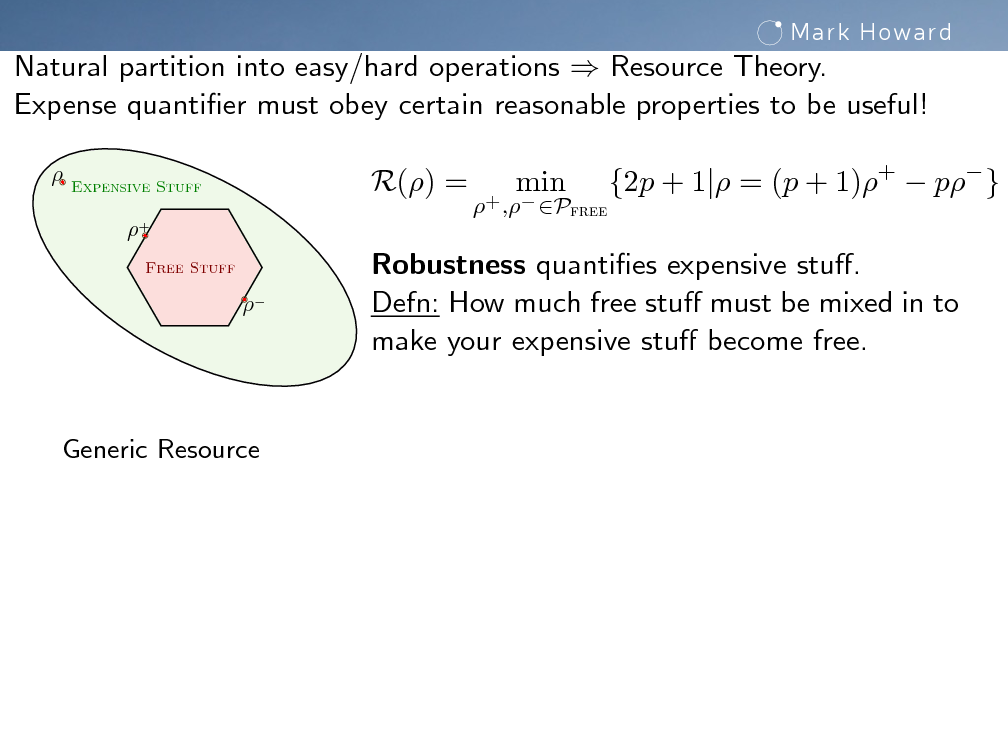
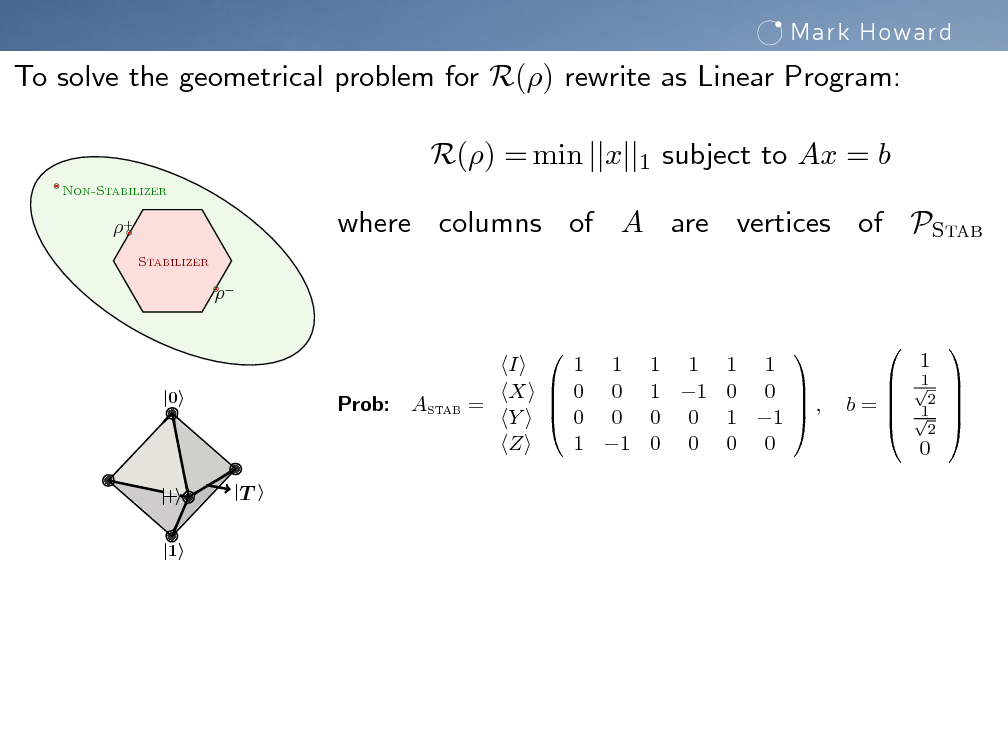
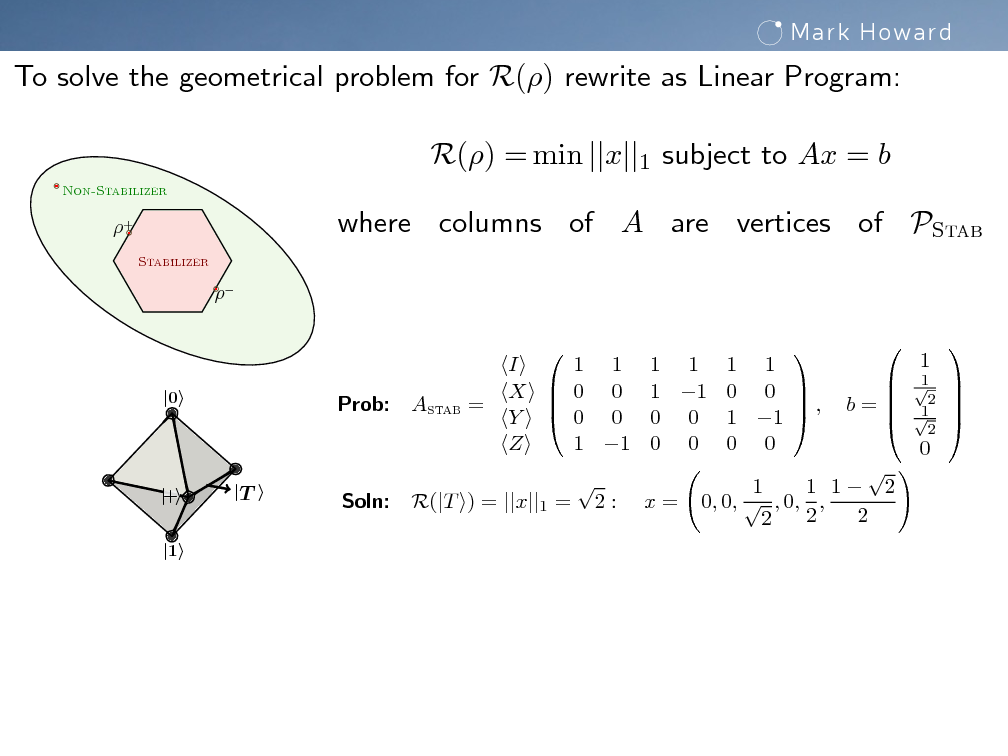
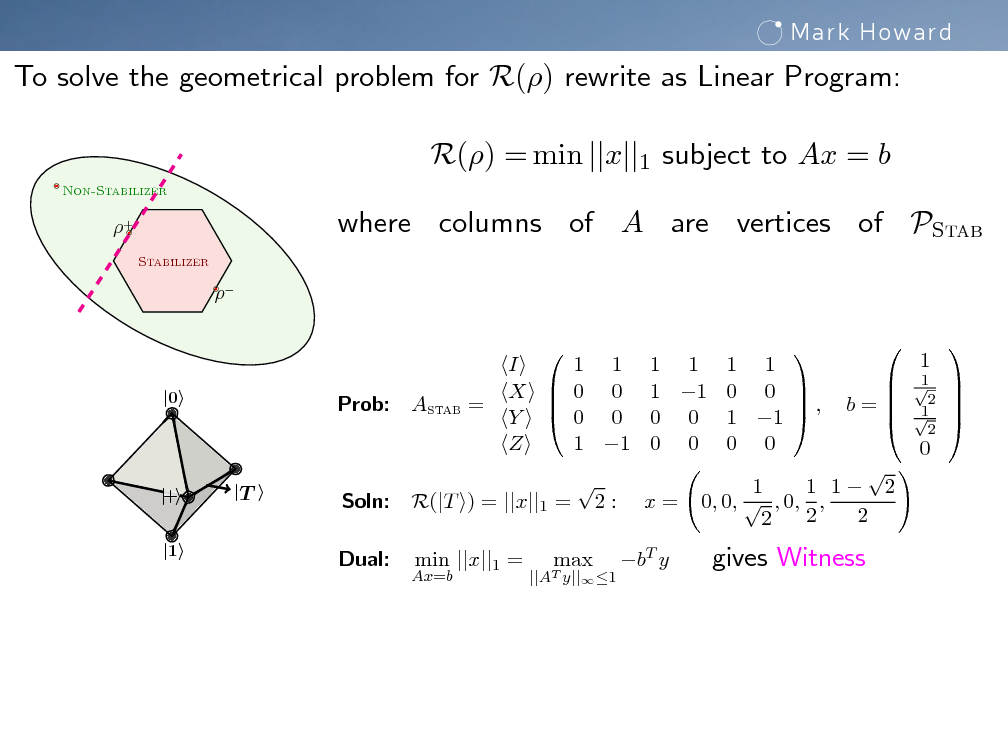
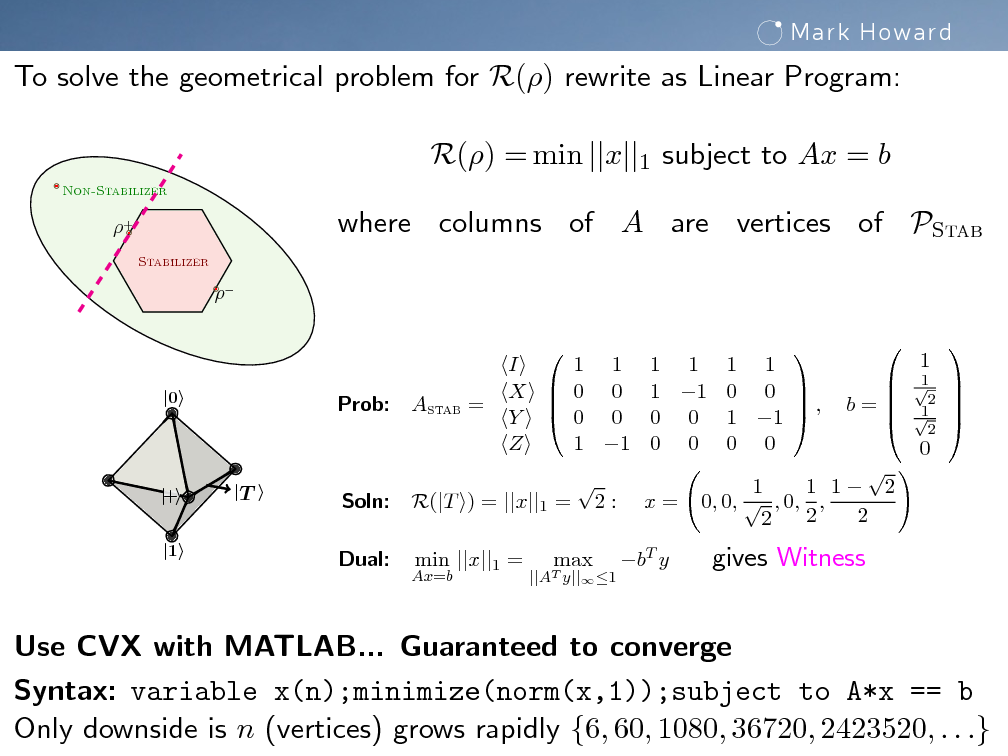
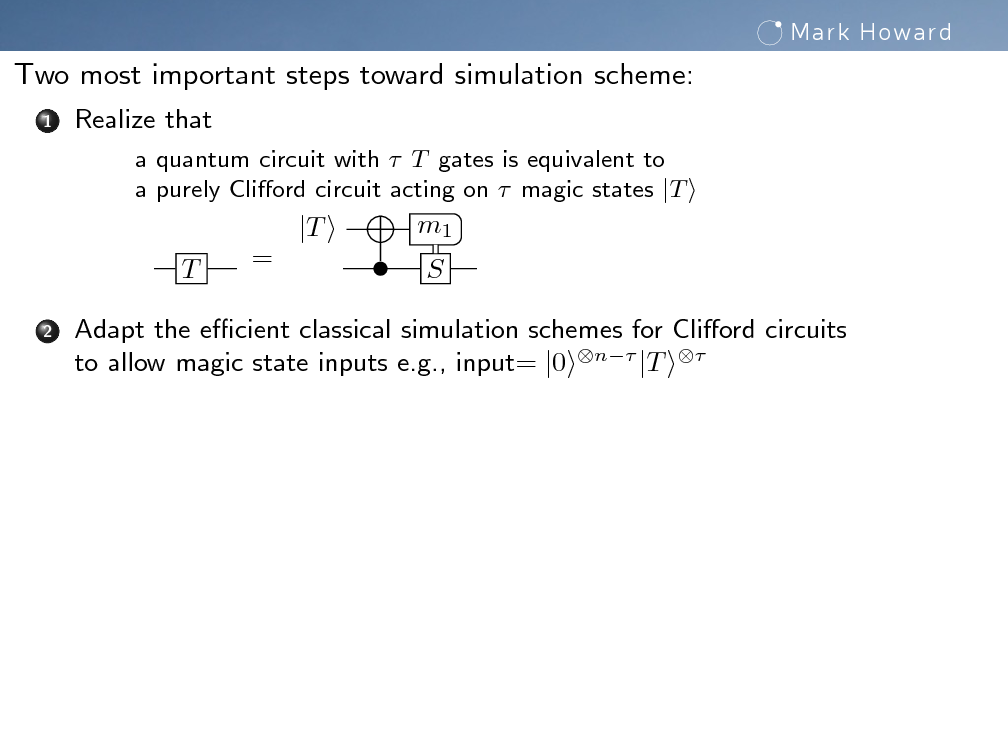
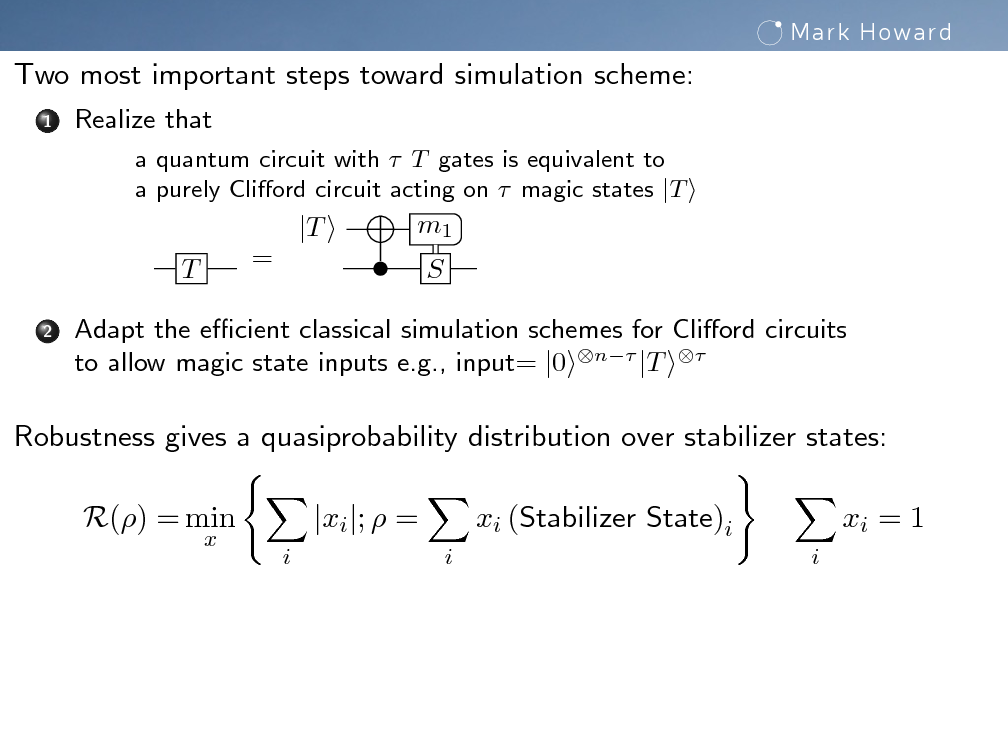
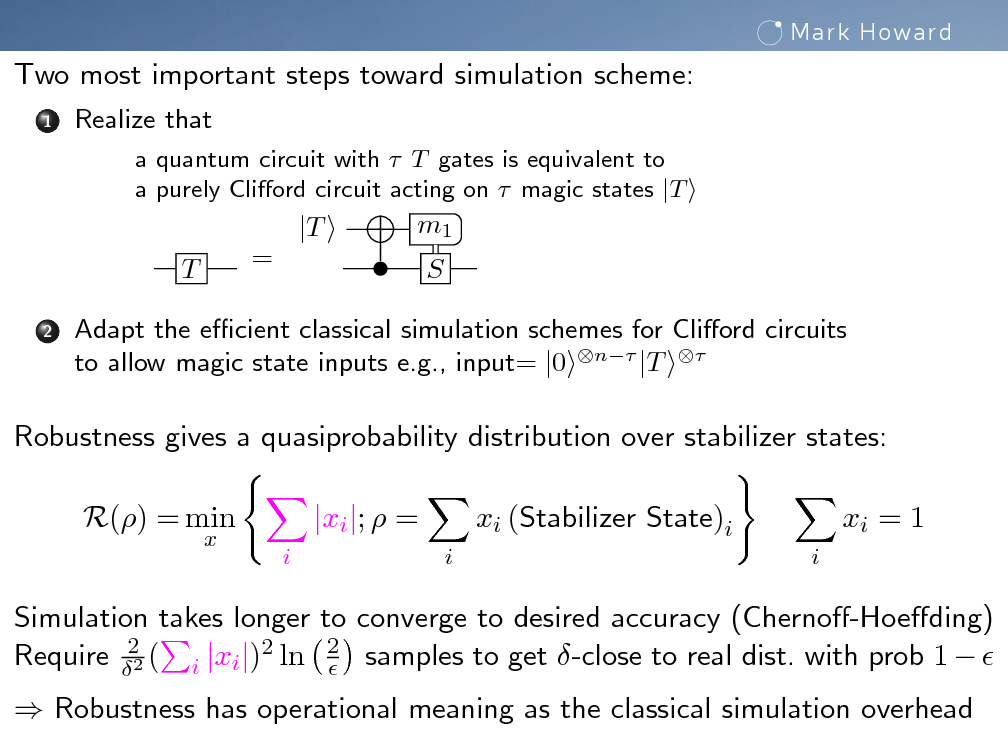
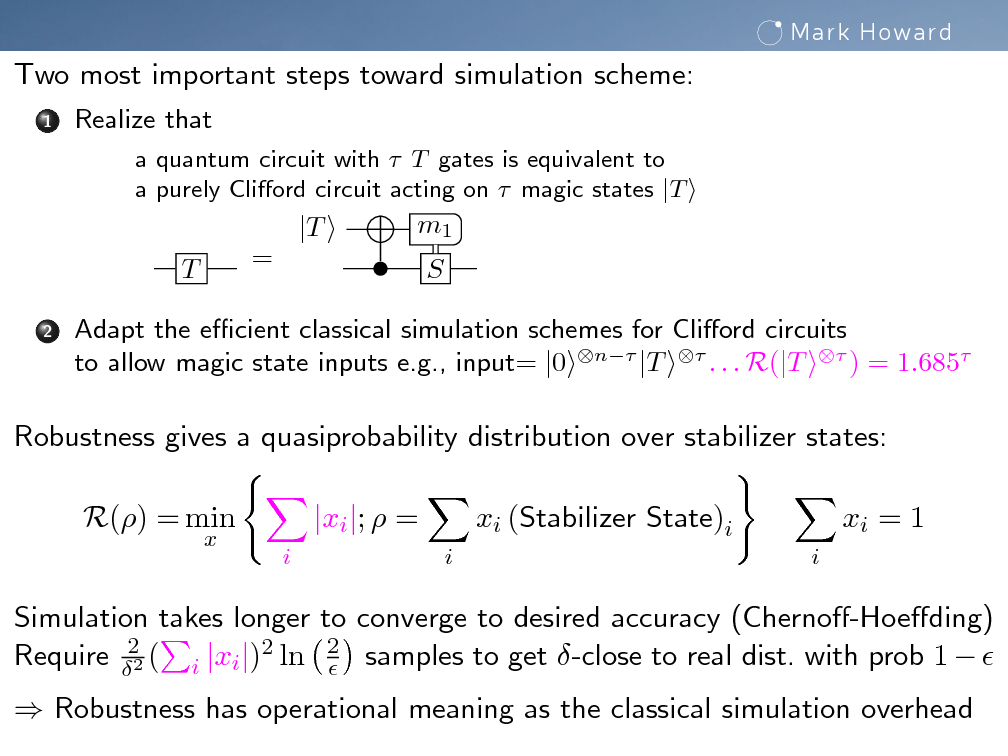
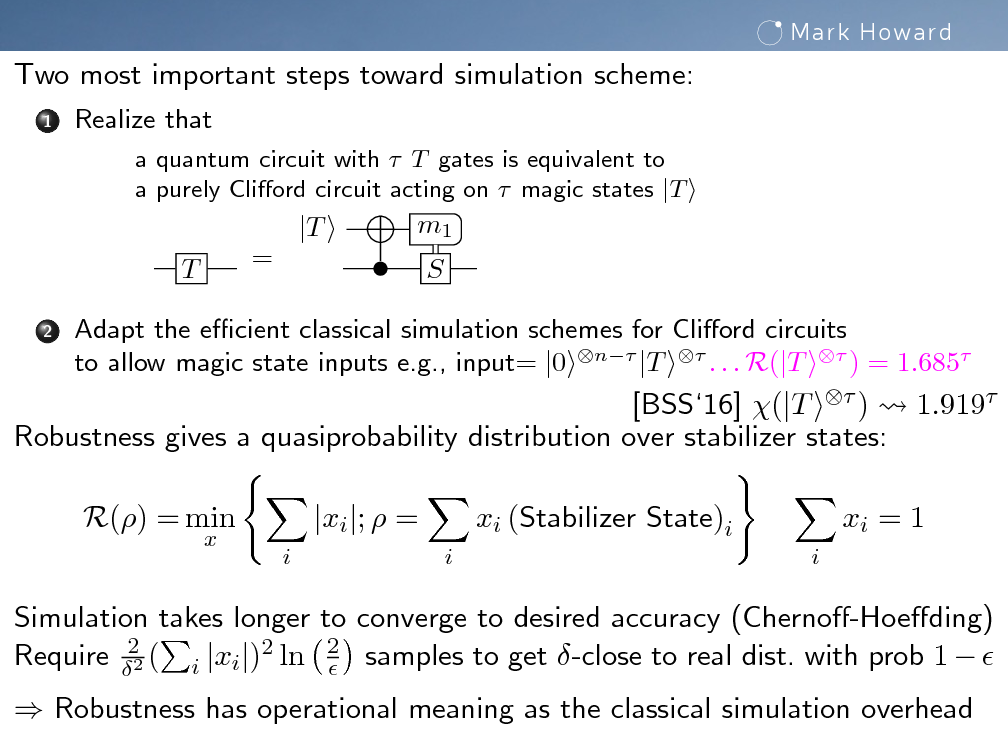
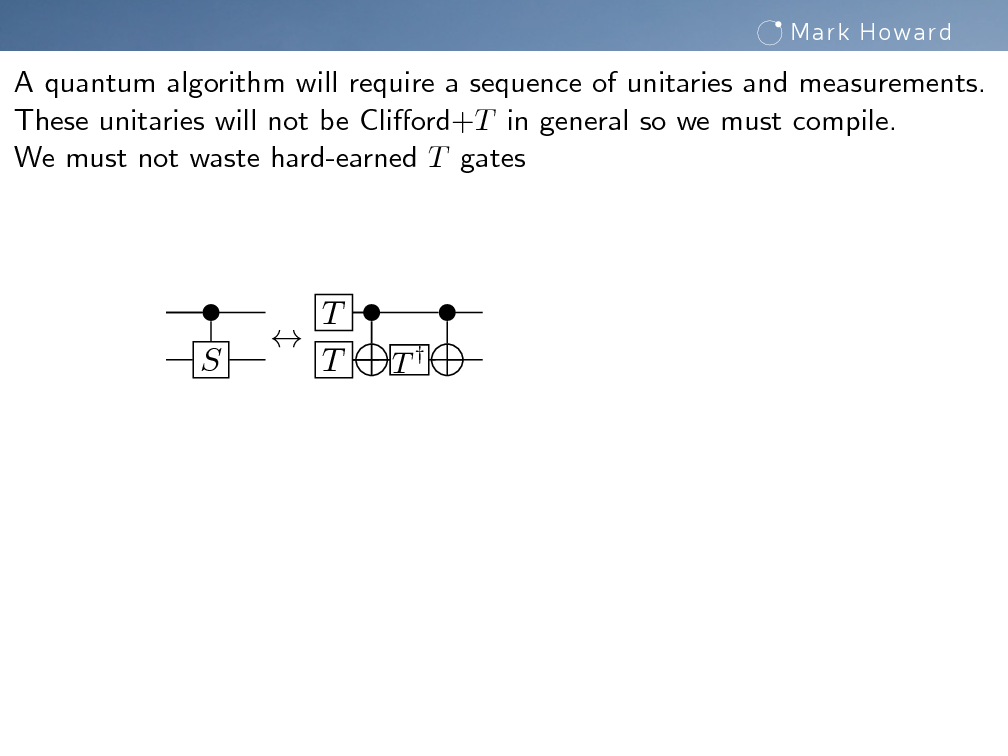
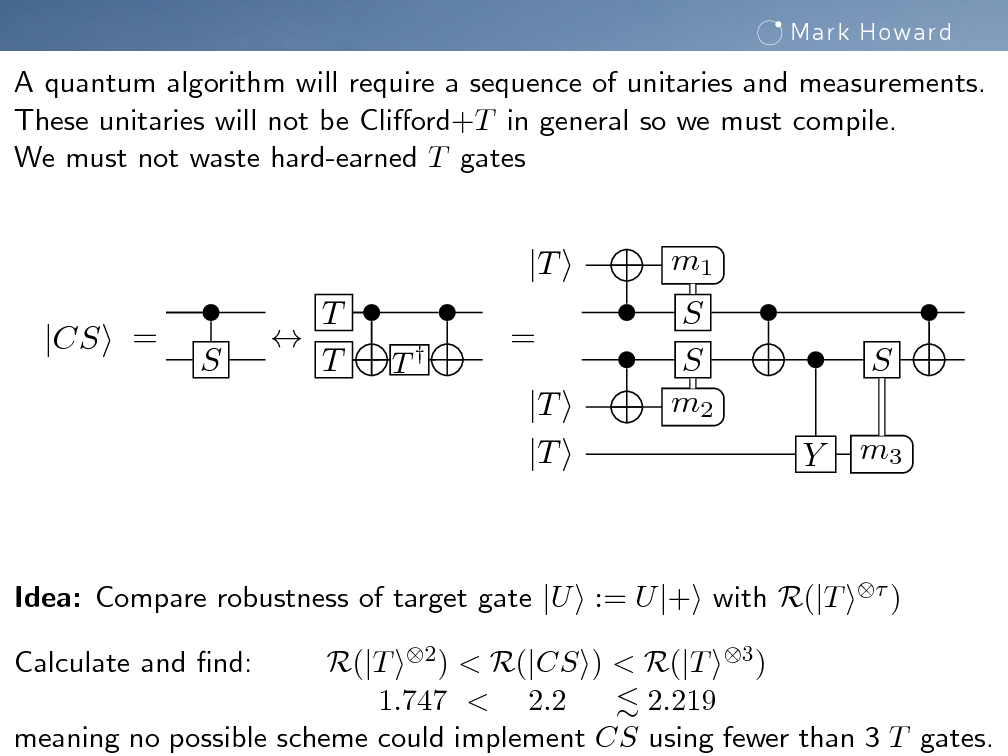
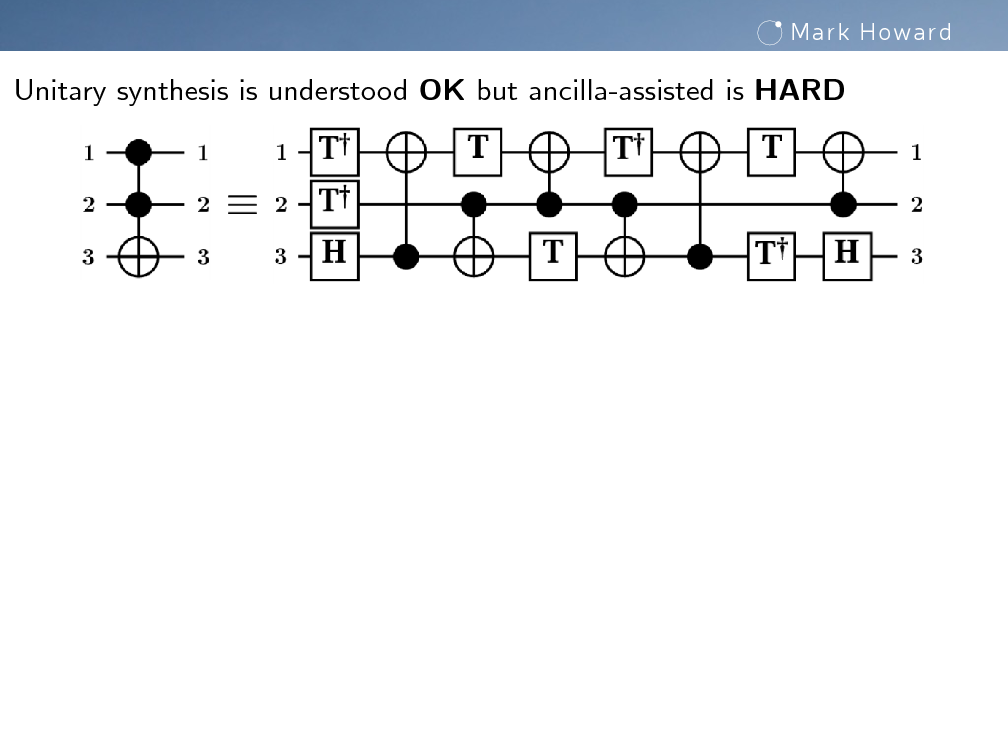
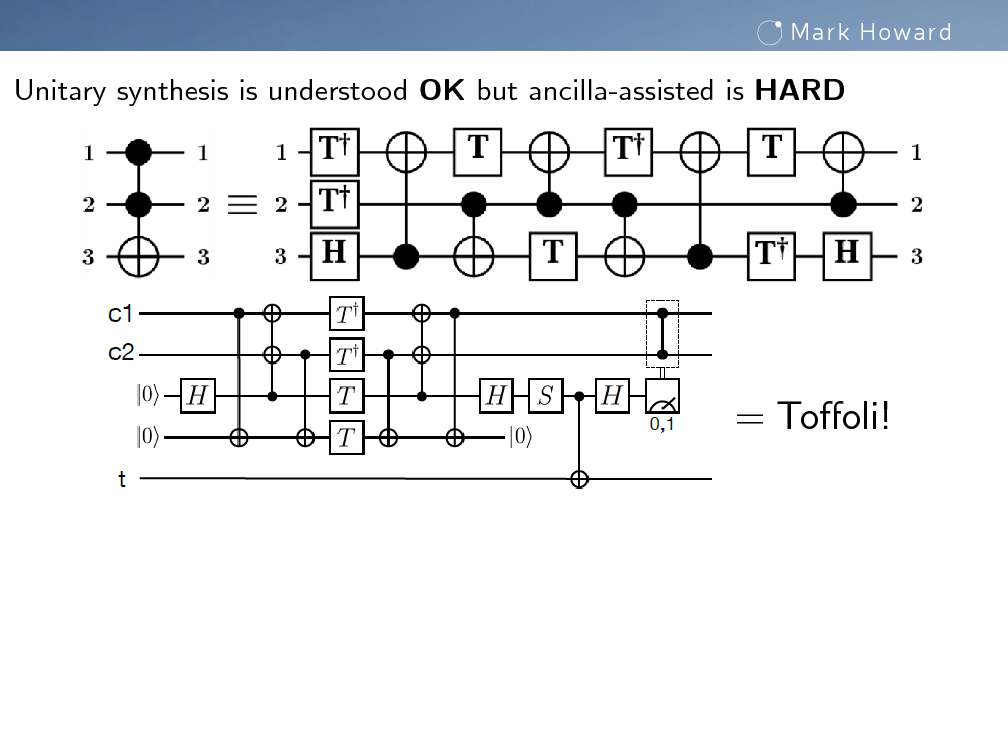
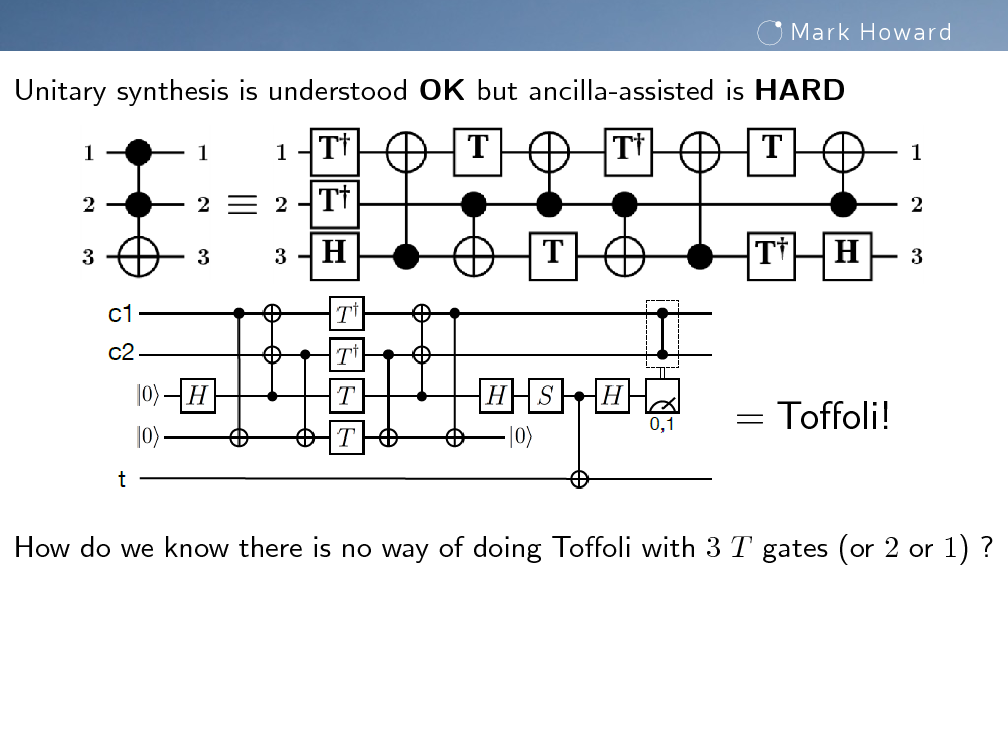
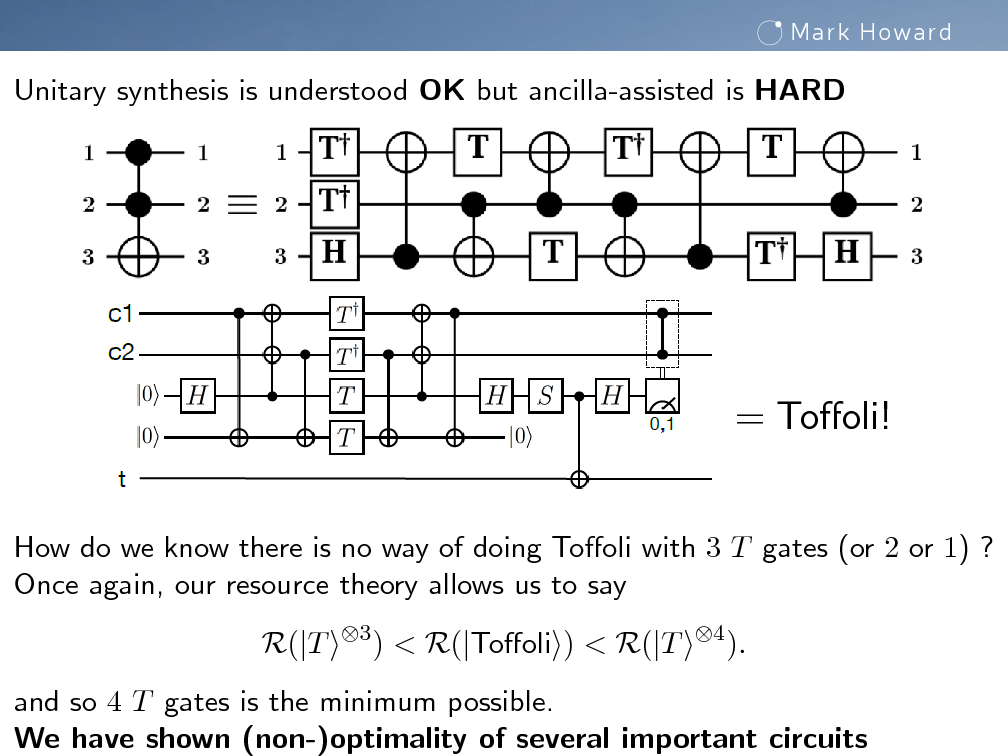
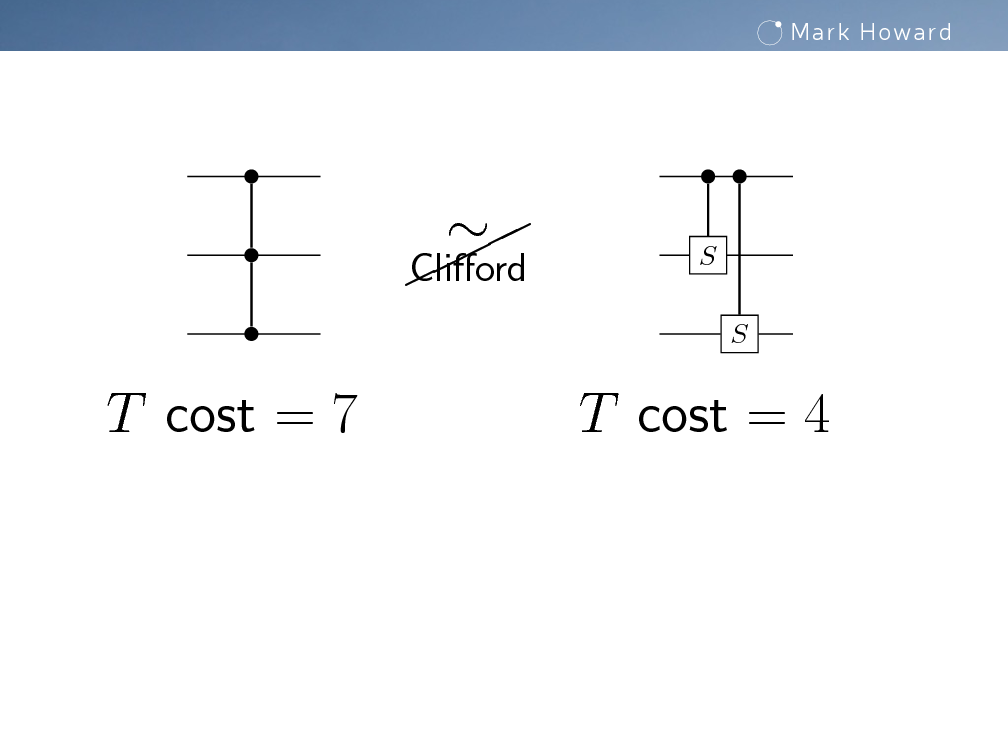
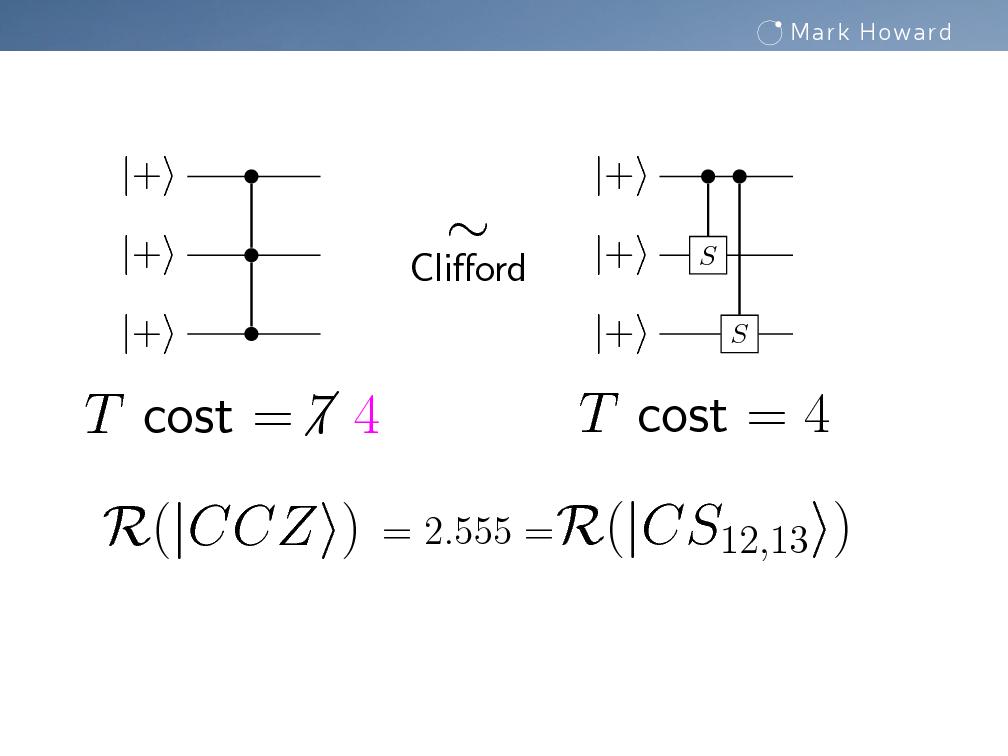
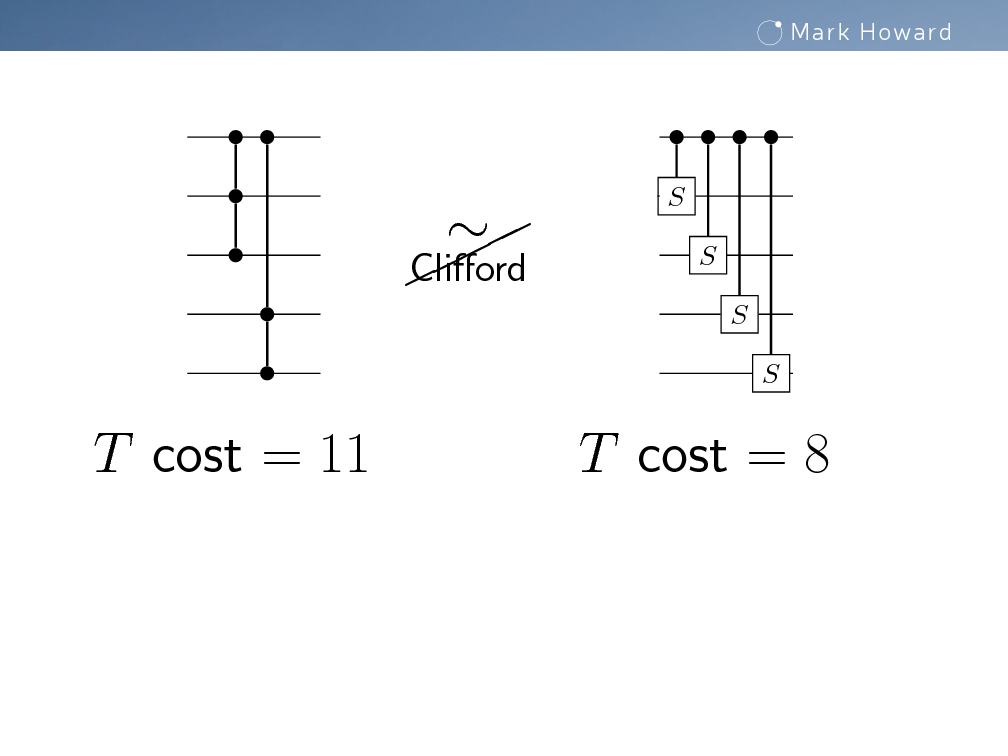
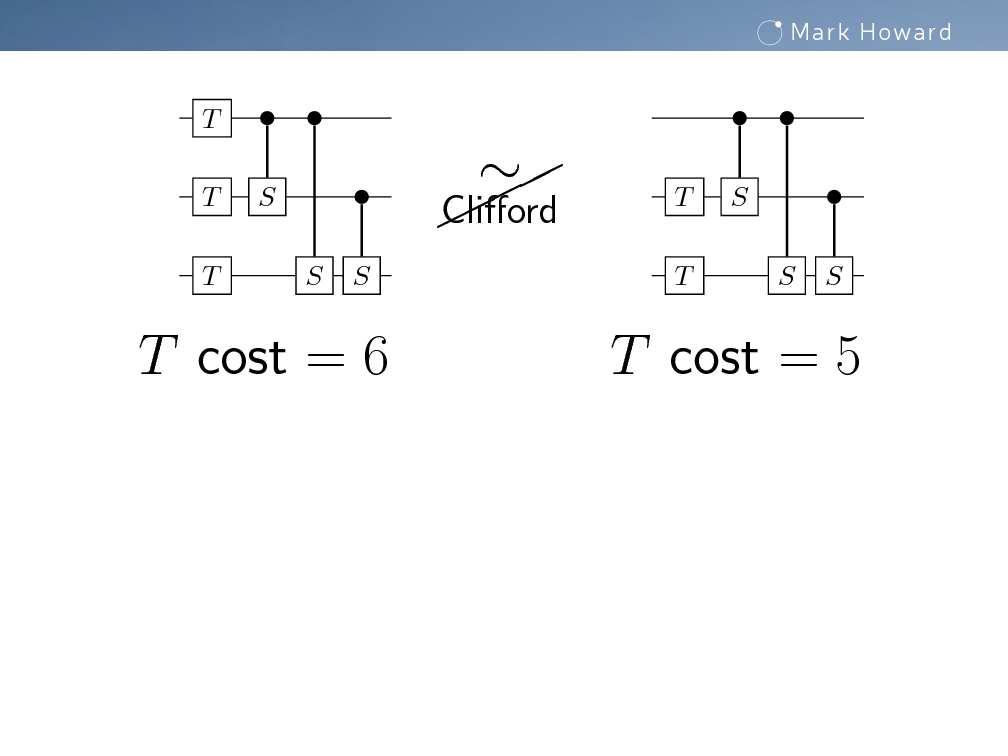
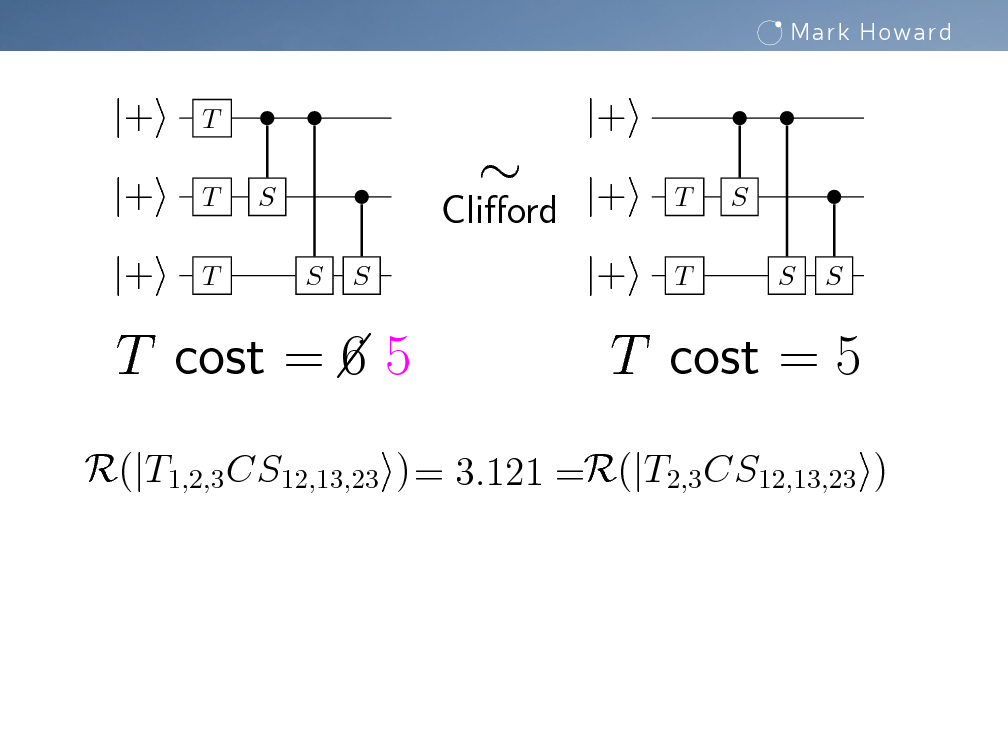
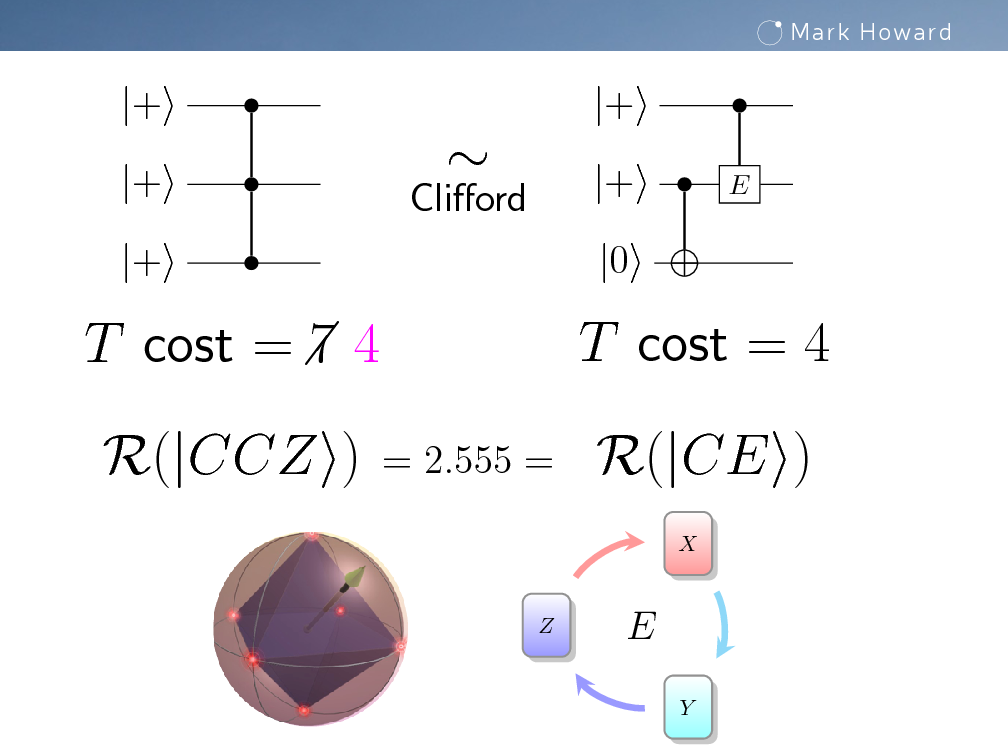
Robustness of Magic

Motivated by their necessity for most fault-tolerant quantum computation schemes, we formulate a resource theory for magic states. We first show that robustness of magic is a well-behaved magic monotone that operationally quantifies the classical simulation overhead for a Gottesman-Knill type scheme using ancillary magic states. Our framework subsequently finds immediate application in the task of synthesizing non-Clifford gates using magic states. When magic states are interspersed with Clifford gates, Pauli measurements and stabilizer ancillas - the most general synthesis scenario - then the class of synthesizable unitaries is hard to characterize. Our techniques can place non-trivial lower bounds on the number of magic states required for implementing a given target unitary. Guided by these results we have found new and optimal examples of such synthesis.